2.E: Gases (Exercises)
2.1 Characteristics of Gases
For a substance that has gas, liquid, and solid phases, arrange these phases in order of
a) increasing density
b) strength of intermolecular interactions
c) compressibility
d) molecular motion.
- Answer
-
a) gas < liquid < solid
b) gas < liquid < solid
d) solid < liquid < gas
d) solid < liquid < gas
2.2 Gas Pressure
Why are sharp knives more effective than dull knives (Hint: think about the definition of pressure)?
- Answer
-
The cutting edge of a knife that has been sharpened has a smaller surface area than a dull knife. Since pressure is force per unit area, a sharp knife will exert a higher pressure with the same amount of force and cut through material more effectively.
Barometric pressure in Vancouver BC was 102.3 kPa. Calculate this barometric pressure in atm and mm Hg.
- Answer
-
1.010 atm, 767.6 mmHg
The barometric pressure at the top of Sun Peaks ski hill near Kamloops BC was 655 mm Hg. What is this pressure in atm and kPa?
- Answer
-
0.861 atm, 87.2 kPa
Canadian tire pressure gauges are marked in units of kilopascals. What reading on such a gauge corresponds to 32 psi?
- Answer
-
2.2 × 102 kPa
The pressure of the atmosphere on the surface of the planet Venus is about 88.8 atm. Compare that pressure in psi to the normal pressure on earth at sea level in psi.
- Answer
-
Earth: 14.7 lb in–2; Venus: 13.1× 103 lb in−2
In November 2021 BC was hit by tremendous storms including an atmospheric river. Between Nov. 13 and Nov. 15 2021 219 mm of rain fell in Chilliwack BC and the barometric pressure dropped from 102.77 kPa to 99.21 kPa. What is the pressure drop in torr?
- Answer
-
26.7 torr
The pressure of a sample of gas is measured at sea level with a closed-end manometer as shown in the figure below. The liquid in the manometer is mercury. Determine the pressure of the gas in:
- torr
- Pa
- bar
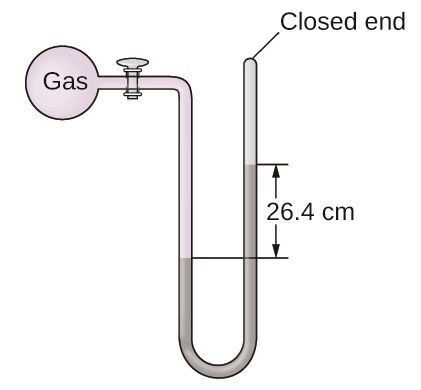
- Answer
-
a. 264 torr; b. 35,200 Pa; c. 0.352 bar
The pressure of a sample of gas is measured at sea level with an open-end mercury manometer. Assuming atmospheric pressure is 760.0 mm Hg, determine the pressure of the gas in:
- mm Hg
- atm
- kPa
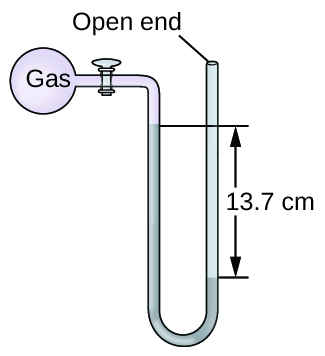
- Answer
-
a. 623 mm Hg; b. 0.820 atm; c. 83.1 kPa
2.3: Relating Pressure, Volume, Amount, and Temperature: The Ideal Gas Law
When a scuba diver exhales gas bubbles, the bubbles rise through the column of water going from higher to lower pressure. Explain how the volume of the bubbles change as they rise to the surface, assuming that they remain intact.
- Answer
-
As the bubbles rise, the pressure decreases, so their volume increases as suggested by Boyle’s law.
An alternate way to state Avogadro’s law is “All other things being equal, the number of molecules in a gas is directly proportional to the volume of the gas.”
- What is the meaning of the term “directly proportional?”
- What are the “other things” that must be equal?
- Answer
-
a. The number of particles in the gas increases as the volume increases. b. temperature, pressure
A spray can which has a pressure of 1344 torr at 23°C is used until it is empty except for the propellant gas. If the can is thrown into a fire at 475°C, what will be the pressure in the hot can in atmospheres?
- Answer
-
4.47 atm
Calculate the volume of 1.00 mol of CH4 gas at 150.0 K and 1.00 atm
- Answer
-
12.3 L
What is the temperature of an 11.2-L sample of carbon monoxide, CO, at 744 torr if it occupies 13.3 L at 55 °C and 744 torr?
- Answer
-
276°K ; 3°C
- Solution
-
we must use [latex]\dfrac{P_1V_1}{T_1} =\dfrac{P_2V_2}{T_2}[/latex] and solve for [latex]T_1[/latex]
[latex]T_1 = \dfrac{P_1V_1T_2}{P_2V_2}[/latex]
Where:
[latex]P_1 = 744\: torr[/latex]
[latex]V_1 = 11.2\: L[/latex]
[latex]P_2 = 744\: torr[/latex]
[latex]V_2 = 13.3\: L[/latex]
[latex]T_2 = 328.15°\: K[/latex]
[latex]\dfrac{(744\: torr)(11.2\: L)(328.15°\: K)}{(744\: torr)(13.3\: L)} = 276°\: K[/latex]276°K ; 3°C
A 2.50-L volume of hydrogen measured at –196 °C is warmed to 100 °C. Calculate the volume of the gas at the higher temperature, assuming no change in pressure.
- Answer
-
12.1 L
A weather balloon contains 8.80 moles of helium at a pressure of 0.992 atm and a temperature of 25 °C at ground level. What is the volume of the balloon under these conditions?

- Answer
-
217 L
How many moles of gaseous boron trifluoride, BF3, are contained in a 4.3410-L bulb at 788.0 K if the pressure is 1.220 atm? How many grams of BF3?
- Answer
-
8.190 × 10–2 mol; 5.553 g
A balloon that is 100.21 L at 21 °C and 0.981 atm is released and just barely clears the top of Mount Crumpet in British Columbia. If the final volume of the balloon is 144.53 L at a temperature of 5.24 °C, what is the pressure experienced by the balloon as it clears Mount Crumpet?
- Answer
-
0.644 atm
Iodine, I2, is a solid at room temperature but sublimes (converts from a solid into a gas) when warmed. What is the temperature in a 73.3-mL bulb that contains 0.292 g of I2 vapor at a pressure of 0.462 atm?
- Answer
-
359°K ; 86°C
- Solution
-
Use the equation [latex]PV =nRT[/latex] and solve for [latex]T[/latex]
[latex]T= \dfrac{PV}{nR}[/latex]
2.) convert grams of I2 to moles of I2 and convert mL to L
[latex]0.292g\: \ce{I2}\times \dfrac{1\: mole\: \ce{I2}}{253.8g\: \ce{I2}} = 1.15 \times10^{-3}\: moles\: \ce{I2}[/latex]
[latex]73.3\:mL = 0.0733\:L[/latex]3.) Use these values along with [latex]R= 0.08206\: \dfrac{atm\:L}{mole\:°K}[/latex] to solve for [latex]T[/latex]
[latex]T= \dfrac{(0.462\: \cancel{atm})(0.0733\:\cancel{L})}{(1.15\times10^{-3}\: \cancel{moles})(0.08206\: \dfrac{\cancel{atm}\:\cancel{L}}{\cancel{mole}\:°K})} = 359\: °K[/latex]
359°K ; 86°C
How many grams of gas are present in each of the following cases?
- 0.100 L of CO2 at 307 torr and 26 °C
- 8.75 L of C2H4, at 378.3 kPa and 483 K
- 221 mL of Ar at 0.23 torr and –54 °C
- Answer
-
a. 7.24 × 10–2 g; b. 23.1 g; c. 1.5 × 10–4 g
A cylinder of medical oxygen has a volume of 35.4 L, and contains O2 at a pressure of 151 atm and a temperature of 25 °C. What volume of O2 does this correspond to at normal body conditions, that is, 1 atm and 37 °C?
- Answer
-
5561 L
A 20.0-L cylinder containing 11.34 kg of butane, C4H10, was opened to the atmosphere. Calculate the mass of the gas remaining in the cylinder if it were opened and the gas escaped until the pressure in the cylinder was equal to the atmospheric pressure, 0.983 atm, and a temperature of 27 °C.
- Answer
-
46.4 g
For a given amount of gas showing ideal behavior, draw labeled graphs of:
- the variation of P with V
- the variation of V with T
- the variation of P with T
- the variation of [latex]\dfrac{1}{P}[/latex] with V
- Answer
-
For a gas exhibiting ideal behavior:
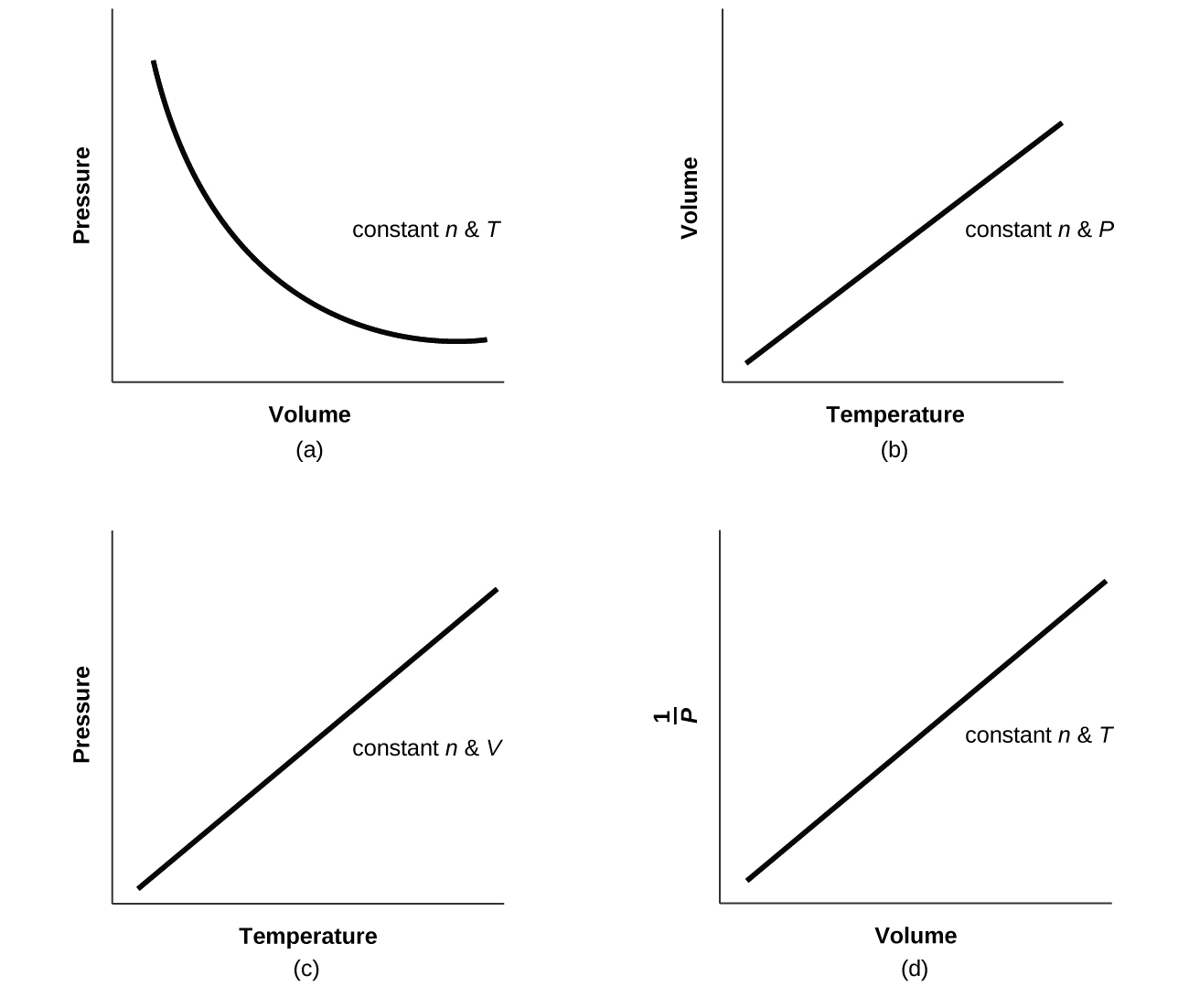
If the volume of a fixed amount of an ideal gas is tripled at constant temperature, what happens to the pressure?
- Answer
-
The pressure decreases by a factor of 3.
Your instructor blows up a balloon so that it is filled with 0.55 moles of Helium at 21.5 oC and 1.00 atm pressure. The instructor adds an additional 0.050 moles of Helium. If the pressure in the balloon remains the same, do you expect the volume to increase or decrease? Explain your reasoning in clear and complete statements.
- Answer
-
Volume is predicted to increase. (explain this answer in your own words)
- Solution
-
under construction
A balloon inflated with three breaths of air has a volume of 1.7 L. At the same temperature and pressure, what is the volume of the balloon if five more same-sized breaths are added to the balloon?
- Answer
-
4.5 L
- Solution
-
[latex]\dfrac{V1}{n1} = \dfrac{V2}{n2}[/latex]
[latex]V2 = \dfrac{V2n2}{n1}[/latex]n1 = 3 breaths = 3x; n2 = (3+5) breaths = 8x
[latex]V2 = \dfrac{(1.7 L)(8x)}{3x}[/latex]
V2 = 4.5 L
- Printable Worksheet
Your Instructor blows up a balloon so that it is filled with 506 mL of Helium at 22.00 oC and 1.00 atm pressure. What volume (in mL) will the balloon have at 4.00 oC?
- Answer
-
475 mL
- Solution
-
under construction
2.4: Stoichiometry of Gaseous Substances, Mixtures, and Reactions
What is the density of laughing gas, dinitrogen monoxide, N2O, at a temperature of 325 K and a pressure of 113.0 kPa?
- Answer
-
1.84 g/L
- Answer
-
First convert kPa to atm
[latex]113.0\:kPa\times\dfrac{1\:atm}{101.325\:kPa}=1.115\:atm[/latex]
2.) The use the equation [latex]d=\dfrac{PM}{RT}[/latex] where d = density in g L-1 and M = molar mass in g mol-1
[latex]d=\dfrac{(1.115\:atm)(44.02\dfrac{g}{\cancel{mol}})}{(0.08206\: \dfrac{\cancel{atm}\:L}{\cancel{mole}\:\cancel{°K}})(325\:\cancel{°K})}=1.84\:\dfrac{g}{L}[/latex]
Calculate the density of Freon 12, CF2Cl2, at 30.0 °C and 0.954 atm.
- Answer
-
4.64 g/L
What is the molar mass of a gas if 0.0494 g of the gas occupies a volume of 0.100 L at a temperature 26 °C and a pressure of 307 torr?
- Answer
-
30.0 g/mol
- Solution
-
Convert torr to atm and °C to °K
[latex]307\:torr=0.404atm[/latex]
[latex]26°C= 300.°K[/latex]2.) Use the equation [latex]PV=nRT[/latex] and solve for [latex]n[/latex]
[latex]n=\dfrac{PV}{RT}[/latex]
[latex]n=\dfrac{(0.404\:\cancel{atm})(0.100\:\cancel{L})}{(0.08206\dfrac{\cancel{atm}\:\cancel{L}}{mol\:\cancel{°K}})(300.\cancel{°K})}=0.00165\:moles[/latex]3.) Then divide grams by the number of moles to obtain the molar mass:
[latex]\dfrac{0.0494g}{0.00165\:moles}=30.0\dfrac{g}{mole}[/latex]
What is the molar mass of a gas if 0.281 g of the gas occupies a volume of 125 mL at a temperature 126 °C and a pressure of 777 torr?
- Answer
-
72.0 g/mol
The density of a certain gaseous fluoride of phosphorus is 3.93 g/L at 0.00 oC and 1.00 atm. Calculate the molar mass of this fluoride and determine its molecular formula.
- Answer
-
88.1 g mol−1; PF3
A 36.0–L cylinder of a gas used for calibration of blood gas analyzers in medical laboratories contains 350 g CO2, 805 g O2, and 4,880 g N2. At 25 degrees C, what is the pressure in the cylinder in atmospheres?
- Answer
-
141 atm
A sample of gas isolated from unrefined petroleum contains 90.0% CH4, 8.9% C2H6, and 1.1% C3H8 at a total pressure of 307.2 kPa. What is the partial pressure of each component of this gas?
- Hint
-
The percentages given indicate the percent of the total pressure that is due to each component.
- Answer
-
CH4: 276 kPa; C2H6: 27 kPa; C3H8: 3.4 kPa
Most mixtures of hydrogen gas with oxygen gas are explosive. However, a mixture that contains less than 3.0 % O2 is not. If enough O2 is added to a cylinder of H2 at 33.2 atm to bring the total pressure to 34.5 atm, is the mixture explosive?
- Answer
-
Yes
A sample of carbon monoxide was collected over water at a total pressure of 756 torr and a temperature of 18 °C. What is the pressure of the carbon monoxide? The vapour pressure of H2O at 18 oC is 15.5 torr.
- Answer
-
740.5 torr or 741 torr
Joseph Priestley first prepared pure oxygen by heating mercuric oxide, HgO:
[latex]\ce{2HgO}(s)⟶\ce{2Hg}(l)+\ce{O2}(g)[/latex]
- Outline the steps necessary to answer the following question: What volume of O2 at 23 °C and 0.975 atm is produced by the decomposition of 5.36 g of HgO?
- Answer the question.
- Answer
-
a. Determine the moles of HgO that decompose; using the chemical equation, determine the moles of O2 produced by decomposition of this amount of HgO; and determine the volume of O2 from the moles of O2, temperature, and pressure.
b. 0.308 L
The chlorofluorocarbon CCl2F2 can be recycled into a different compound by reaction with hydrogen to produce CH2F2(g), a compound useful in chemical manufacturing:
[latex]\ce{CCl2F2}(g)+\ce{4H2}(g)⟶\ce{CH2F2}(g)+\ce{2HCl}(g)[/latex]
- Outline the steps necessary to answer the following question: What volume of hydrogen at 225 atm and 35.5 °C would be required to react with 1 ton (1.000 × 103 kg) of CCl2F2?
- Answer the question.
- Answer
-
a. Determine the molar mass of CCl2F2. From the balanced equation, calculate the moles of H2 needed for the complete reaction. From the ideal gas law, convert moles of H2 into volume.
b. 3.72 × 103 L
Lime, CaO, is produced by heating calcium carbonate, CaCO3; carbon dioxide is the other product.
- Outline the steps necessary to answer the following question: What volume of carbon dioxide at 875° and 0.966 atm is produced by the decomposition of 1 ton (1.000 × 103 kg) of calcium carbonate?
- Answer the question.
- Answer
-
a. Balance the equation. Determine the grams of CO2 produced and the number of moles. From the ideal gas law, determine the volume of gas.
b. 7.43 × 105 L
Calculate the volume of oxygen required to burn 12.00 L of ethane gas, C2H6, to produce carbon dioxide and water, if the volumes of C2H6 and O2 are measured under the same conditions of temperature and pressure.
- Answer
-
42.00 L
Consider the following questions:
- What is the total volume of the CO2(g) and H2O(g) at 600 °C and 0.888 atm produced by the combustion of 1.00 L of C2H6(g) measured at 0.00 oC and 1.00 atm?
- What is the partial pressure of H2O in the product gases?
- Answer
-
a. 18.0 L
b. 0.533 atm
What volume of oxygen at 423.0 K and a pressure of 127.4 kPa is produced by the decomposition of 129.7 g of BaO2 to BaO and O2?
- Answer
-
10.57 L O2
Ethanol, C2H5OH, is produced industrially from ethylene, C2H4, by the following sequence of reactions:
[latex]\ce{3C2H4 + 2H2SO4⟶C2H5HSO4 + (C2H5)2SO4}[/latex]
[latex]\ce{C2H5HSO4 + (C2H5)2SO4 + 3H2O⟶3C2H5OH + 2H2SO4}[/latex]
What volume of ethylene at 0.00 oC and 1.00 atm is required to produce 1.000 metric ton (1000 kg) of ethanol if the overall yield of ethanol is 90.1%?
- Answer
-
5.40 × 105 L
A sample of a compound of xenon and fluorine was confined in a bulb with a pressure of 18 torr. Hydrogen was added to the bulb until the pressure was 72 torr. Passage of an electric spark through the mixture produced Xe and HF. After the HF was removed by reaction with solid KOH, the final pressure of xenon and unreacted hydrogen in the bulb was 36 torr. What is the empirical formula of the xenon fluoride in the original sample? (Note: Xenon fluorides contain only one xenon atom per molecule.)
- Answer
-
XeF2
2.5: The Kinetic-Molecular Theory
A 1-L sample of CO initially at 0 oC and 1 atm is heated to 546 K, and its volume is increased to 2 L.
- What effect do these changes have on the number of collisions of the molecules of the gas per unit area of the container wall?
- What is the effect on the average kinetic energy of the molecules?
- What is the effect on the root mean square speed of the molecules?
- Answer
-
a. The number of collisions per unit area of the container wall is constant.
b. The average kinetic energy doubles.
c. The root mean square speed increases to [latex]\sqrt{2}[/latex] times its initial value; urms is proportional to [latex]\mathrm{KE_{avg}}[/latex].
The distribution of molecular velocities in a sample of helium is shown in Figure 2.5.4. If the sample is cooled, will the distribution of velocities look more like that of H2 or of H2O? Explain your answer.
- Answer
-
H2O. Cooling slows the velocities of the He atoms, causing them to behave as though they were heavier.
Calculate the root-mean-square speed of nitrogen, N2, molecules in an air sample at 25 oC.
- Answer
-
515 m/s
What is the ratio of root-mean-square speed values for helium to xenon at 30 oC? Which has a higher root-mean-square speed and why?
- Answer
-
0.18, so He has a higher root-mean-square speed
He has a higher root-mean-square speed than xenon, He should be faster as is has a smaller molar mass.
- Hint
-
The temperature is the same so the ratio of root-mean-square speeds becomes the square root of the ratio of molar masses.
-

2.6: Effusion and Diffusion of Gases
A balloon filled with helium gas is found to take 6 hours to deflate to 50% of its original volume. How long will it take for an identical balloon filled with the same volume of hydrogen gas (instead of helium) to decrease its volume by 50%?
- Answer
-
4.2 hours
Starting with the definition of rate of effusion and Graham’s finding relating rate and molar mass, show how to derive the Graham’s law equation, relating the relative rates of effusion for two gases to their molecular masses.
- Answer
-
Effusion can be defined as the process by which a gas escapes through a pinhole into a vacuum. Graham’s law states that with a mixture of two gases A and B: [latex]\mathrm{\left(\dfrac{rate\: A}{rate\: B}\right)=\left(\dfrac{molar\: mass\: of\: B}{molar\: mass\: of\: A}\right)^{1/2}}[/latex]. Both A and B are in the same container at the same temperature, and therefore will have the same kinetic energy:
[latex]\mathrm{KE_A=KE_BKE}=\dfrac{1}{2}mv^2[/latex] Therefore, [latex]\dfrac{1}{2}m_\ce{A}v^2_\ce{A}=\dfrac{1}{2}m_\ce{B}v^2_\ce{B}[/latex] [latex]\dfrac{v^2_\ce{A}}{v^2_\ce{B}}=\dfrac{m_\ce{B}}{m_\ce{A}}[/latex] [latex]\left(\dfrac{v^2_\ce{A}}{v^2_\ce{B}}\right)^{1/2}=\left(\dfrac{m_\ce{B}}{m_\ce{A}}\right)^{1/2}[/latex] [latex]\dfrac{v_\ce{A}}{v_\ce{B}}=\left(\dfrac{m_\ce{B}}{m_\ce{A}}\right)^{1/2}[/latex]
Which of the following gases diffuse more slowly than oxygen? F2, Ne, N2O, C2H2, NO, Cl2, H2S
- Answer
-
F2, N2O, Cl2, H2S
Calculate the relative rate of diffusion of 1H2 (molar mass 2.0 g/mol) compared to that of 2H2 (molar mass 4.0 g/mol) and the relative rate of diffusion of O2 (molar mass 32 g/mol) compared to that of O3 (molar mass 48 g/mol).
- Answer
-
1.4; 1.2
2.7: Non-Ideal Gas Behavior
Under which of the following sets of conditions does a real gas behave most like an ideal gas, and for which conditions is a real gas expected to deviate from ideal behavior? Explain.
- high pressure, small volume
- high temperature, low pressure
- low temperature, high pressure
- Answer
-
The gas behavior most like an ideal gas will occur under the conditions in (b). Molecules have high speeds and move through greater distances between collision; they also have shorter contact times and interactions are less likely. Deviations occur with the conditions described in (a) and (c). Under conditions of (a), some gases may liquefy. Under conditions of (c), most gases will liquefy.
For which of the following gases should the correction for the molecular volume be largest: CO, CO2, H2, He, NH3, SF6?
- Answer
-
SF6
Answer the following questions:
- If XX behaved as an ideal gas, what would its graph of Z vs. P look like?
- For most of this chapter, we performed calculations treating gases as ideal. Was this justified?
- What is the effect of the volume of gas molecules on Z? Under what conditions is this effect small? When is it large? Explain using an appropriate diagram.
- What is the effect of intermolecular attractions on the value of Z? Under what conditions is this effect small? When is it large? Explain using an appropriate diagram.
- In general, under what temperature conditions would you expect Z to have the largest deviations from the Z for an ideal gas?
- Answer
-
a. A straight horizontal line at 1.0
b. When real gases are at low pressures and high temperatures they behave close enough to ideal gases that they are approximated as such, however, in some cases, we see that at a high pressure and temperature, the ideal gas approximation breaks down and is significantly different from the pressure calculated by the van der Waals equation
c. The greater the compressibility, the more the volume matters. At low pressures, the correction factor for intermolecular attractions is more significant, and the effect of the volume of the gas molecules on Z would be a small lowering compressibility. At higher pressures, the effect of the volume of the gas molecules themselves on Z would increase compressibility (see Figure 2.7.1)
d. Once again, at low pressures, the effect of intermolecular attractions on Z would be more important than the correction factor for the volume of the gas molecules themselves, though perhaps still small. At higher pressures and low temperatures, the effect of intermolecular attractions would be larger. (see Figure 2.7.1)
e. low temperatures
A 0.245-L flask contains 0.467 mol CO2 at 159 °C. Calculate the pressure:
(a) using the ideal gas law
(b) using the van der Waals equation
(c) Explain the reason for the difference.
- Answer
-
a. 67.6 atm
b. 60.5 atm
c. The van der Waals equation pressure is lower than the ideal gas pressure because at these conditions the molecules are attracted to each other so are applying less force on the walls of the flask and the molecules take up space so the volume available to the gas is less than the flask volume. The van der Waals equation corrects for the intermolecular attractions and the volume of gas molecules.
